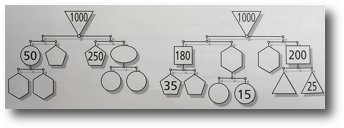
Designing mobiles
You could make a mobile using card shapes, wooden dowels (or straws) and cotton to demonstrate how they balance. However, to show how they work with the complementary pairs of numbers it is helpful to draw a simple shape mobile like this on the board.
Starting point
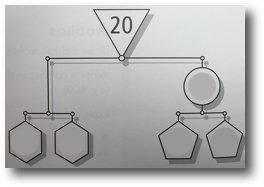
Write a number in the triangle, for example 20.
KS1 can find complements to 10 and 20, KS2 can find complements of 100 and1000 as well as all the decade numbers to 100.
Explain that:
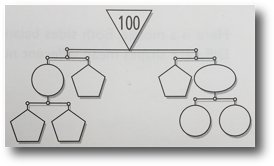
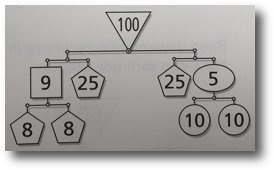
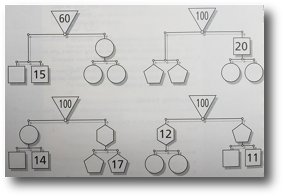
Shapes that are the same contain the same number.
Each side of a balance must have the same total.
Ask for different possible solutions.
Practising complementary pairs
‘Show me…’ type activities with children using sets of arrow cards or fan numbers to hold up the answers are useful for practicing these skills:
The total is 20. Show me the number that must be added to 12, 9, 16, 5…
The total is 80. Show me the number that must be added to 50, 20, 72, 25…
The total is 100. Show me the number that must be added to 30, 60, 45, 76…
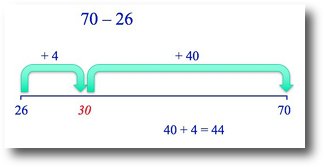
Using a number line for this helps children to visualise the jumps that the complementary addition involves.
It is an important way for children to represent their mental process, the step before children progress on to formal written methods of subtraction.
26 + __ = 30
30 + __ = 70
If we unpick this skill a little more, it is clear that children need to have quick recall of the complements of 10, 100 and 1000 as well as the decade numbers.