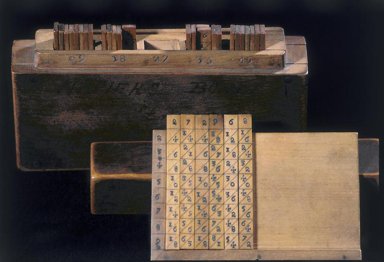
The Science Museum has a new maths collection and one of the artefacts is a wooden set of Napier’s ‘Bones’, you can visit the new maths collection online. Set your class the challenge of discovering how to multiply large numbers using Napier's numbers on a grid. A great problem solving activity that holds children's interest while reinforcing quick recall of mulitplication facts.
The Science Museum opened the Winton Gallery for mathematics in December. It spans 400 years of mathematical history and shows how mathematics has shaped our world. The collection online can be explored by children without direction, so they can follow their own interests, alternatively you can choose an item and bring it into your teaching.
There are lots of things to explore online and my favourite is the wooden set of Napier’s Bones c. 1690. A great starting point is to get your class to explore how to use these ‘bones’ to multiply large numbers.
There are lots of things to explore online and my favourite is the wooden set of Napier’s Bones c. 1690. A great starting point is to get your class to explore how to use these ‘bones’ to multiply large numbers.
Explore Napier's Bones
Make you own bones
The best way to get children to understand how the ‘bones' work is to get them to make their own.
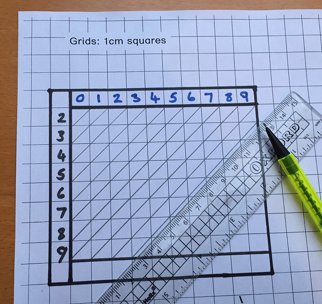
• Use 1cm square grid paper and draw a grid, 11 x 9 squares
• Write the numbers 0-9 along the top and the numbers 2-9 down the left-hand side.
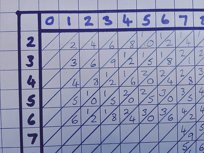
• Draw diagonal lines as shown
• Explain that numbers above the diagonal lines are ‘tens’ and below the lines are ‘ones’
• Multiply the top number by the side number and write in the answer with tens written in the top corner and ones written in the bottom corner.
• Cut the strips into columns leaving the side attached to a bottom strip.
You have now made your own set of Napier's bones.
Set the task
Use the Napier's Bones activity on NRICH - print out the NRICH page and give as a worksheet.
It is always good to give children the chance to discover for themselves. Working in pairs and groups works well, but for this type of activity I find it better to give children the chance to work on their own and then share their findings with others. Also remember the importance of thinking time and try not to rush children to find the solution.
The first task is to work out how to find the answer to 24 x 6 using Napier’s Bones. Then the subsequent multiplications are more difficult. In 8931 x 6, children will have to consider what to do with answers in the diagonals that go over 10.
Teacher Prompts
There are some things you can do to help if they need a nudge in the right direction:
• Use a calculator to find the actual answer - this might help them see how the numbers on Napier’s Bones could give the same answer.
• Look at the photographs of the wooden Napier’s Bones on the Science Museum website. There are a few clues in the pictures about how they may have been used.
• Place the strips next to the side grid, column of 2 and column of 4, beside each other to make 24 across the top.
• Remind children that when they are investigating they will get stuck – and this is an ‘honourable state’ as it means they are having to think really hard and mathematically. Once they stop being stuck, they will get an ‘AHA!’ moment.
As a teacher it is always a good idea to complete the task yourself - you’ll find the places that you get stuck and how the progression in the task leads on to higher level maths thinking.
There are some things you can do to help if they need a nudge in the right direction:
• Use a calculator to find the actual answer - this might help them see how the numbers on Napier’s Bones could give the same answer.
• Look at the photographs of the wooden Napier’s Bones on the Science Museum website. There are a few clues in the pictures about how they may have been used.
• Place the strips next to the side grid, column of 2 and column of 4, beside each other to make 24 across the top.
• Remind children that when they are investigating they will get stuck – and this is an ‘honourable state’ as it means they are having to think really hard and mathematically. Once they stop being stuck, they will get an ‘AHA!’ moment.
As a teacher it is always a good idea to complete the task yourself - you’ll find the places that you get stuck and how the progression in the task leads on to higher level maths thinking.
Related articles
Calculator challenges - a useful tool in the primary classroom
Exploring multiplication patterns using a calculator.
Testing times tables - balance it with a mulitplcation games
Download and print a game to practise quick recall of multiplcation facts
Grouping and multiplcation - is 2 x 3 two 3s or three 2s?
If it is 'groups of' then it is 3+3, but if it is 'multiplied by' it is 2+2+2
What do your calss think? Which should you teahc?
Calculator challenges - a useful tool in the primary classroom
Exploring multiplication patterns using a calculator.
Testing times tables - balance it with a mulitplcation games
Download and print a game to practise quick recall of multiplcation facts
Grouping and multiplcation - is 2 x 3 two 3s or three 2s?
If it is 'groups of' then it is 3+3, but if it is 'multiplied by' it is 2+2+2
What do your calss think? Which should you teahc?